ANALYTIC GEOMETRY - GOOD PROBLEMS from Alex Pintilie

Together:

 

1)a) Consider the fixed points A(-a,0) and B(a,0). Find the equation of the locus of the points M with the property MA2 - MB2 = k, where k is a constant. Identify the locus.

b) Consider the points A (-2, 0) B(2,0). Find the equation of the locus of the points M with the property MA2 - MB2 = 12. Draw a diagram.

 

2)a) Consider the fixed points A(-a,0), B(a,0). Find the equation of the locus of the points M with the property MA = k MB, where k is a constant. Identify the locus.

b) Consider the fixed points A(-4,0), B(4,0). Find the equation of the locus of the points M with the property MA = 2 MB. Draw a diagram.

3) Consider the concentric circles with equations  and . A radius from the centre O intersects the inner circle at P and the outer circle at Q. The line parallel to the x-axis through P meets the line parallel to the y-axis through Q at the point R. Prove that R lies on the ellipse .

Class work:

 

1) Let P be a variable point on the line x=1. We construct the triangle OPQ with O the origin, ŠO=90o, OP=OQ. Find the locus of the point Q.

 


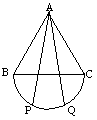
2) For the equilateral triangle ABC, side BC is the diameter of a semicircle.

 Points P and Q create equal arcs BP = PQ = QC on the semicircle. Show that line

 segments AP and AQ trisect side BC. (Hint: express P and Q using polar coordinates)

 

 

Homework:

 

1)a) Consider the fixed points A(a,-b) and B(a,b). Find the equation of the locus of the points M with the property MA2 - MB2 = k, where k is a constant. Identify the locus.

b) Consider the points A (3, -6) B(3,6). Find the equation of the locus of the points M with the property MA2 - MB2 = 12. Draw a diagram.

 

2)a) Consider the fixed points A(a,-b), B(a,b). Find the equation of the locus of the points M with the property MA = k MB, where k is a constant. Identify the locus.

b) Consider the fixed points A(4,6), B(4,-6). Find the equation of the locus of the points M with the property MA = 3 MB. Draw a diagram.

 

3) Two trees stand at A and B, a considerable distance apart in the middle of a flat plain. The tree at B is twice the height of the tree at A. There is a road on the plane and, as I cycle along it I am struck by the fact that the two trees appear to be exactly the same size. As the road moves towards them, the trees both appear to get larger, but they continue always to look the same size as one another. What is the shape of the road? Using a clearly marked system of axis, graph A, B and the road. (Answer: The shape is a circle.)

 

4) The point M “travels” on the fixed segment AB. Construct the squares AMEF and BMDC on the same side of the segment AB. Prove that the position of the midpoint P of the segment FC does not depend on the position of the point M on AB.

 

5) The line with equation y = x - 2 intersects the circle with equation  at the points A and B, with B in the first quadrant. At B a line is drawn perpendicular to the given line to meet the circle again at point C. Determine the area of the triangle ABC.  (Answer: Area = 7)

 

MORE ANALYTIC GEOMETRY

 

1) Consider the points A(-2,0), B(4,0), C(6,0). Through C we draw a (variable) line which intersects the line y=x at M and the y-axis at N. Find the locus of the intersection of the lines AM and NB. (Hint: Write the equation of the variable line as y = k(x-6) with k variable. Express M and N in terms of k. Find the coordinates of the intersection of AM and NB in terms of k. Eliminate k between the two coordinates. The locus will be a line.)

 

2) Given the parabola,   (), consider the line l  of slope m=1 which passes through the variable point M (a,0). The line and parabola intersect at points A and B, |AB|£.

a)       Find the possible range of values for a.

b)       The perpendicular bisector of AB intersects theaxis at the point N, find the area of the triangle NAB.