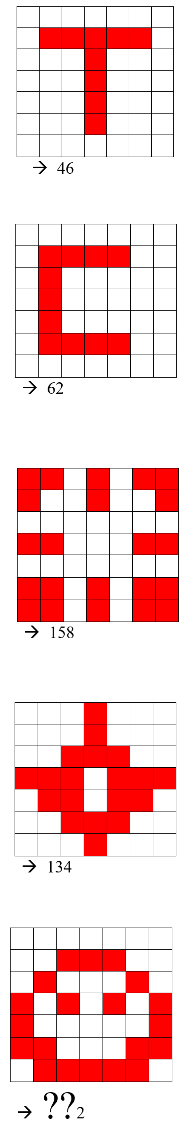
Can you determine the missing two digits of the three-digit number for the last diagram?
Note: I struggled with this problem and was not able to solve it, but I know many of you are smarter than I am,
so I decided to post it. I have included a hint (which I was not afforded), so if you would like the hint,
scroll down ...
You must use PRIME NUMBERS to solve the problem.
Also, it has nothing to do with different number bases (of which I tried many).
Solution:
The two missing digits are 1 and 4, so the number is 142 (you were given the 2).
To get the number for each diagram, perform the following steps:
Count the number of red squares.
Then find the prime number corresponding to it.
For example, if there were 7 red squares, determine the 7th prime number which is 17.
Multiply the prime number by two to get the answer.
In diagram 1:
There are 9 red squares.
The ninth prime number is 23.
Multiply by 2 to get 46.
In diagram 2:
There are 11 red squares.
The 11th prime number is 31.
Multiply by 2 to get 62.
In diagram 3:
There are 22 red squares.
The 22nd prime number is 79.
Multiply by 2 to get 158.
In diagram 4:
There are 19 red squares.
The 19th prime number is 67.
Multiply by 2 to get 134.
In diagram 5:
There are 20 red squares.
The 20th prime number is 71.
Multiply by 2 to get 142.
Correctly solved by:
| 1. Kamal Lohia |
Holy Angel School, Hisar, Haryana, India |
| 2. K. Sengupta | Calcutta, India |
| 3. Kelly Stubblefield | Mobile, Alabama, USA |
| 4. Pam Slone | Thacker, West Virginia, USA |