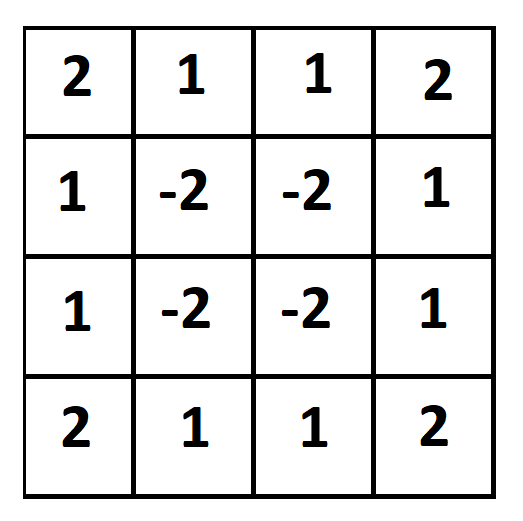
An integer is written on each unit square of a 4×4 unit square chessboard.
The number in each unit square is equal to the sum of the numbers in all unit squares that share a common edge with that unit square.
What is the largest number of positive integers (out of the 16 numbers) that can be written on this chess board?