
(1) Determine the area of each of the four original shapes.
(A) Right Triangle: _______
(B) Right Triangle: _______
(C) Trapezoid: ____________
(D) Trapezoid: ____________
(2) Determine the area of each of the figures that you formed
with the four shapes:
(A) Square: _______________
(B) Rectangle: ____________
(C) Triangle: _____________
(D) Trapezoid: ____________
(E) Octagon: ______________
(F) Parallelogram: ________
(G) Another Triangle: _____
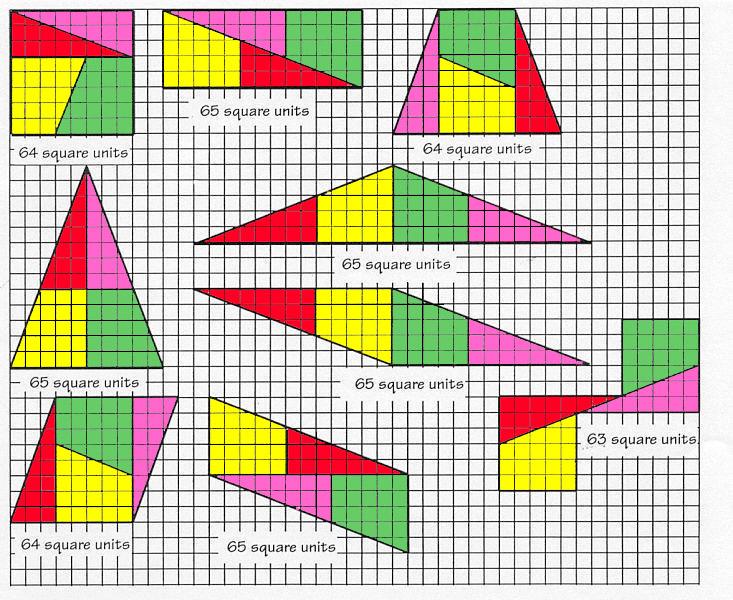
There appears to be a paradox! Some of the figures have 63
square units, some have 64 square units, and some have 65 square units.
This seems paradoxical since all the figures were formed with the same
four pieces.
Here are some solutions for the figures above:
How do you explain this impossible situation?
Take a closer look at the first two figures — the square and the rectangle.
The four pieces fit snugly together for the square.
The area of the square is 64 square units, which makes sense since the
areas of the four smaller pieces are 12 square units, 12 square units,
20 square units, and 20 square units.
The area of the rectangle is 65 square units, but where does that extra
square unit come from?
Look at the diagram below and you will notice that the pieces do not fit
snugly together in the rectangle (the extra square inch can be seen
in the middle).
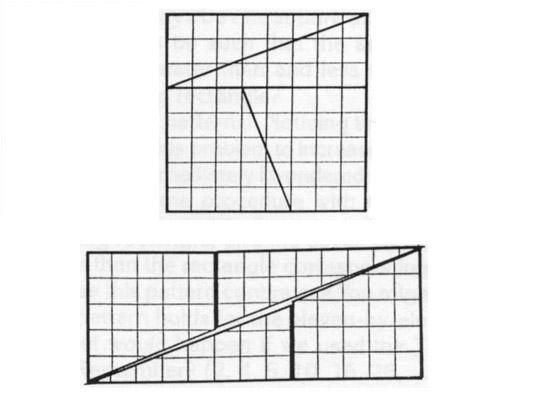
How can you prove this?
If the pieces indeed did fit together to form a rectangle, then
the diagonal would be one line segment. If it is just one segment,
then it would have just one slope, since the slope of a line is always
the same. Determine the slopes of the
four pieces that form "the" diagonal (the slopes should be 2/5, 2/5, 3/8,
and 3/8). Notice that they are not the same.
Therefore, it is not one segment (which can be seen in the drawing above).
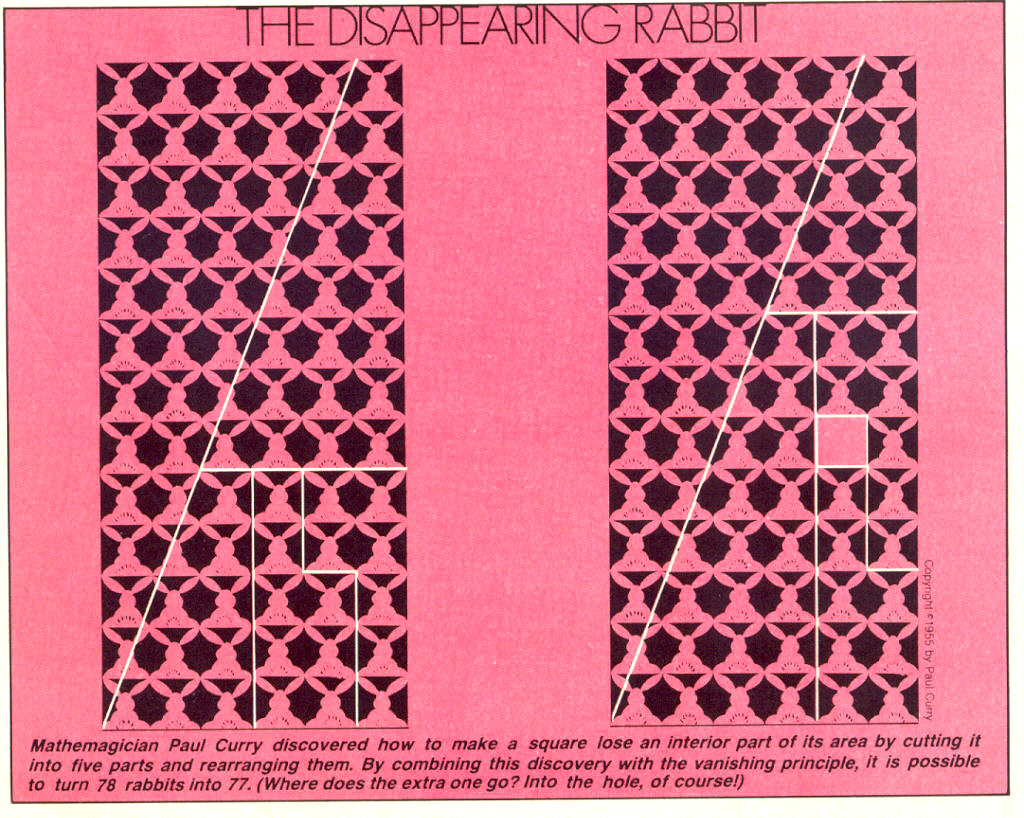
Here is how one magician used this paradox for a magic trick:
Here is a video of this paradox.