There is a mathematical curve that is brought to mind by fads in general. It is the S-shaped curve. This curve characterizes, or at least seems to characterize, a variety of phenomena, including the demand for new toys. Its shape can most easily be explained by imagining a few bacteria in a petri dish (see diagram). At first, the number of bacteria will increase at a rapid exponential rate because of the rich nutrient broth and the ample space in which to expand. Gradually, however, as the bacteria crowd each other, their rate of increase slows and the number of bacteria stabilizes.
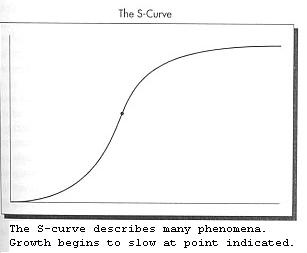
Interestingly, this curve {sometimes called the logistic curve) appears to describe the growth of entities as disparate as Mozart's symphony production, the rise of airline traffic, new mainframe computer installations, and the building of Gothic cathedrals. If you can't think of more, the writer Cesare Marchetti and others have amassed a large number of them and speculated that there is a kind of universal principle governing many natural and human phenomena. What is especially provocative about the supposition is that in many of their examples, there doesn't seem to be anything analogous to the nutrients in the petri dish -- no resources whose depletion leads to an end to exponential growth and a gradual leveling off.
But there is something that is being continuously depleted with time, and that is the {admittedly vague) sense of novelty. Our natural proclivity to focus on the unusual, the dramatic, and the new is strengthened immeasurably by newspapers and other media, but our interest wanes quickly as well. We're so fascinated by the sudden rise of the new celebrity, the spread of the titillating rumor, and the increasingly frequent accounts of some exotic condition or lurid crime that we forget the trivial fact that many phenomena have a limited life span. And given this life span, it shouldn't be too surprising if some phenomenon begins small, takes off, and then gradually tapers off. It might be interesting to plot the cumulative number of mentions that previously unknown persons, ideas, or fads receive in, say, the New York Times to determine which ones generate S-curves.
The mathematics of the S-curve cannot be predictive of these phenomena without more precise information. It may be nothing more than a suggestive mathematical metaphor. Supplementing it with plausible empirical assumptions about the curve's parameters and arguments about its applicability, biologists have accurately predicted the growth of bacteria in limited environments. Human population experts have also used it to forecast that the world's population will level off at 11 to 12 billion.
We've all heard the television anchor with the authoritative voice intoning that such and such an index is declining (or rising) or seen the generic headline proclaiming THINGS GETTING WORSE (or BETTER). When are such pronouncements warranted? At what point can we say, for example, that we are witnessing a deterioration? Is it when the index is falling or when its ascent is slowing or its descent quickening? Arguments can be adduced for the last two positions. (In calculus, the question is whether the first derivative is negative -- the index is falling -- or whether the second derivative is negative -- either the ascent is slowing or the descent is quickening.) The point is that even in this very simple case, a mere decline in the relevant index needn't be a cause for despair nor need a rise be a cause for joy.
The point on the graph of the S-curve where it switches from being concave up (smiling) to concave down (frowning) is a critical one. This is where the growth, though still positive, begins to slow. If the quantity indexed is something desirable, then, in a sense, things start to get worse at this point. In another sense, they're just getting better more slowly. We must examine what the specific index in question is measuring to evaluate the situation.
One last point about these indices: Situations in which the value of such an index is rising most rapidly are often only superficially worrisome (or hopeful, depending upon what is being measured). An example is the increase in the incidence of AIDS in rural women over sixty years of age. If the baseline incidence is very low, a few new cases can result in stories announcing a dramatic rise in the relevant index.
Rates of change, rates of rates of change, and the relations among them constitute the bulk of the mathematical discipline of differential equations. It's noteworthy that the rudiments of the subject are implicit in seemingly straightforward news stories. Continuing on this topic, however, will lead us beyond the point where the S-curve of interest in it begins to level off.